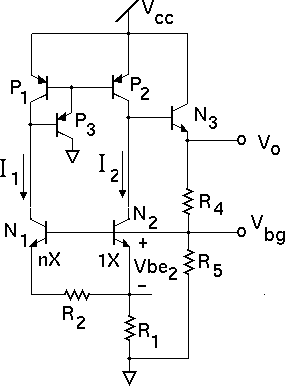
Figure 1 Band gap voltage reference outout is independent of temperature and Vcc.
 Figure 1 Band gap voltage reference outout is independent of temperature and Vcc.
|
VT is proportional to temperature. Vbe decreases with temperature at about -2mV/oC. The first term in equation 2 increases with temperature and the second term decreases with temperature. These changes compensate each other when changes with temperature are minimized. Taking the derivative of equation 2 with respect to temperature and setting it equal to zero and rearranging terms,where VT = KT/q = 0.026V at T = 300 oK
I1 = n Is exp[Vbe1/VT] = I2 = Is exp[Vbe2/VT]
n exp[Vbe1/VT] = exp[Vbe2/VT] VTln[n] = Vbe2 - Vbe1 = R2I1
I = I1 = VT ln[n]/R2
(1) Vbg = R12 I + Vbe2
Vbg = R12 VTln[n]/R2 + Vbe2
(2) VT = 8.62 X 10-5T
2 (R1/R2) ln[n] = .002/8.62 X 10-5ExampleR1/R2 = 11.6/ln[n]
If n = 4
R1/R2 = 8.4
If n = 4 the voltage across R2 is about 36mV. If R2 equals 450 ohms, I will equal 80 uA and R1 should equal about 3.7K. The drop across R1 is 2 I R1 = 2*80X10-6*3.7X103 = 0.6V.The bandgap voltage, Vbg, is 0.6 + Vbe2 = 0.6 + 0.65 = 1.25V